Summary

The present study reports the results obtained by simulating a rotary piercing process. In particular, the mechanism of hole formation and propagation for seamless tube production has been modelled via FEM simulation and the outputs have been compared with the experimental evidence.

The first phase of the research is focused on the definition of a reliable model and on its implementation into a two-dimensional simulation code. The technological data characterising the productive process have been defined in cooperation with Pietra S.p.A..

The second phase consists in the simulation of the start and propagation of the hole and in the comparison between simulation and experimental results. The utilised simulation code is DEFORM 2D, its remeshing module has been modified to model fracture initiation and propagation, that is start and development of the internal hole.

1. INTRODUCTION

When the need of industries is for tubes, different technology for tube productions can be considered. In particular, depending on the final application, and on the required dimensions, tubes can be manufactured by extrusion, welding or rotary piercing. If the need is for seamless tubes and the ratio length-diameter of the tube is high, the best technology for tube production is represented by the rotary piercing process or Mannesman process.

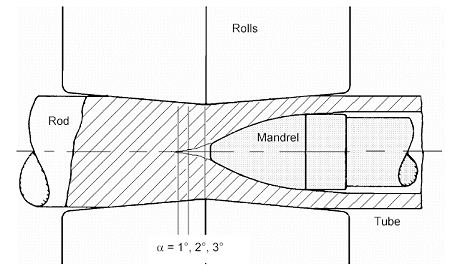
By analysing the rotary tube piercing process as reported in Figure 1, a hole is formed by peripherally rolling a cylindrical hot round bar over a conical piercer point. The rod is driven by a pair of cone-shape rolls which have skewed axis and rotate in the same direction. The frictional load between the rolls and the rod causes the rod to rotate and forces it to advance longitudinally over the piercer point, where the internal fracture starts [1-3].

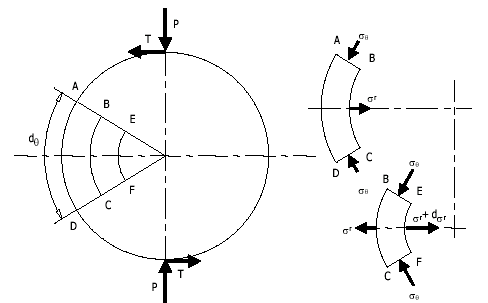
More in detail, the round bar rotates and undergoes to a cyclic progressive compression which results in high tensile stresses in the centre of the rod itself as reported in Figure 2. According to the theory of plasticity fracture starts exactly at the centre of the bar because the tensile stress reaches its breakage value. The internal hole is then sized and calibrated by an internal mandrel (Figure 1).

Figuure 1. The rotary tube piercing process

Figure 2. Stress distribution in the transversal section of the rod

2. RESEARCH AND PRODUCTION

The mechanism of hole formation with a Rotary Piercing Mill represents one of the most important aspects of the productive cycle of steel seamless tubes. This is a subject addressed by many researchers, designers, mill builders and tube makers, who are continuously looking at innovative solutions so to improve the technology of the process itself.

The results gained by the research team of the University of Brescia in cooperation with Pietra S.p.A. company, and reported in the following of this paper, refer to the analysis of stresses generated during the hole formation of a hot round bar by using a rotary piercing mill. These results are relevant for the productive reality and for the future development of the research.

The technical information, achieved through simulations, together with the knowledge on the seamless tubes process formation, can furnish to all the workers of this field useful elements to deeply understand the complex phase of hole formation. This knowledge will give the possibility to optimize some parameters which are governing the productive process. As an example, the working temperature of the round bar, the geometry of the rolls, the shape and the position of the head of the mandrel, and other more. These aspects are all of priority importance to obtain the best qualitative and productive performance.

3. STUDY OF MATERIAL BREAKAGE

The study of material fracture is a very relevant topic. In fact, if production processes involving material breakage, such as for example blanking, cutting or process related to development of internal cracks (extrusion or tube rotary piercing), want to be addressed the simulation program has to deal with modelling of fracture. Fracture can be simulated mainly in two different ways:

1. splitting the mesh nodes,
2. deleting the mesh elements.

The FEM model, used for the simulation of material fracture and hole formation and presented in this paper, is a customised version of DEFORM 2D, a lagrangian implicit code. Material breakage can be simulated by deleting the mesh elements of the workpiece material when the damage value is higher than a defined critical value. Using this code the Authors obtained good results in the study of orthogonal cutting, blanking and forecast of chevron cracks in foreword extrusion [10-12].

The developed model was applied also to rotary tube piercing as reported in [13]. The promising results obtained in the first part of the research gave to the Authors the idea to continue the research itself and to concentrate the efforts in the definition of a model able to match the experimental evidence. Of course, this could be done thanks to the active cooperation and experience of Pietra S.p.A. which since 1946 works in the field of steel seamless tube production by using a rotary piercing mill.

4. THE IMPLEMENTED FRACTURE MODEL

As already mentioned, the developed code is a customisation of the standard DEFORM 2D version. The modified code differs from the original one in the possibility of implementing a new damage criterion (which defines how and when fracture begins), in the subroutines for deleting the elements, and in the remeshing module (which improves the mesh quality after deleting the elements).

To study the material breakage ductile fracture criteria have been used. In fact, the main assumption of these criteria is that ductile fracture occurs when the maximum damage value in the workpiece exceeds a critical value or a so called “critical damage value" (Ccr). The big problem related to this approach is the definition of the critical damage value. In fact, its choice affects when and how fracture begins. As a consequence, its value has to be defined under realistic working conditions, taking into account the effects of working temperature, of strain, strain rate on the flow stress and on the breaking stress.

In previous publications the Authors considered several damage criteria such as, for example, Oyane, 1972, Cockroft and Latham, 1968 and McClintock, 1968 [4-8]. But, what it has been found to give better results is the choice of a criterion able to take into account the state of stresses which develops during the piercing process. 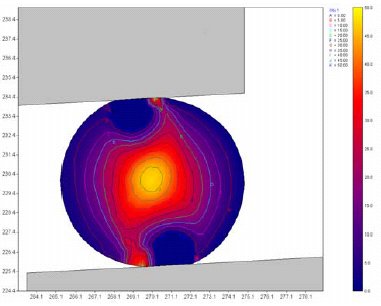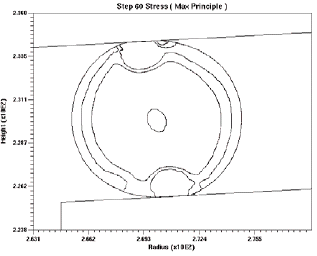In particular, by analysing the mechanism of hole formation and its dependence on the stress state, a criterion based on the Maximum Principal Stress has been implemented. Figure 3 shows the calculated maximum principal stress, it is evident that it reaches the higher value in the centre of the round bar. When the maximum principal stress is higher than a critical value ólim, which is the limit stress, the fracture starts form the centre of the rod. To implement the Maximum Principal stress criterion, the value of maximum principal stress has to be calculated for each element of the mesh according to:

Using the sign convention , the value of the maximum principal stress can be easily calculated:

Figure 3. Plot of the calculated maximum principal stress

5. MODEL DEFINITION AND IMPLEMENTATION

The simulation program utilised is a two-dimensional FEM code. As a consequence, a 2D model has to be realised to represent the deformation process. In the rotary tube piercing process the round bar rotates around its axis, moreover the fracture is located on a plane perpendicular to the rotation axis of the round bar. Considering these two assumptions, only the circular section of the rod has been taken into account.

The simulation model in the deformation area is a plain strain. The progressive compression force exerted by the rolls which determines the rotation and the translation of the bar has been modelled using dies with different inclination so behaving as the conical rolls (Figure 4). The designed dies move along opposite orizontal direction and with a velocity equal to the radial velocity of the rolls in the contact area between rolls and bar.

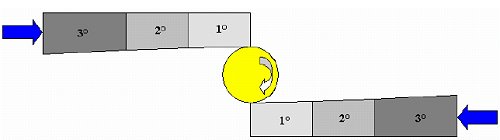
To simulate the movement of the rod in the rolling direction, the dies have been divided in three sections as reported in Figure 1, namely á 1°, 2° and 3°. The realised model can study the rotation and the translation in the rolling direction of the rod, as the rod advances the inclination angle of the dies increases and the compressive force increases as well. In this way, it is possible to consider the deformation history of the bar and to understand its influence on flow stress and on the mechanism of fracture formation.

Figure 4. The simplified two dimensional model

6. THE SIMULATIONS

The geometry of the process has been defined in cooperation with a company (PIETRA SpA) which produces seamless tubes by using a rotary tube piercing mill. In particular, a round bar of 150 mm diameter, made of AISI 1020 steel at a working temperature of 1250° C, has been modelled. The rotation speed of the cylinders is 80 rpm and the cylinder diameter is 780 mm.

Three transversal sections of the rod have been simulated, the corresponding die is characterised by three different inclination angles of 1°, 2°, and 3° degree respectively (Figure 1and 4).

The simulation mode is plane strain and isothermal, that means that the effect of temperature is neglected. The round bar material is plastic and the flow stress of the AISI 1020 steel has been found in literature [9], the dies are considered perfectly rigid. Friction at the contact between rod and rolls has been modelled as shear with a constant friction factor m = 0.8. The high value of friction factor represents the productive reality. In fact, the high friction is determined by the difficulty of lubrication and by the high temperature of the bar and to the very high roughness of the rolls in the compressive area. To model the hole formation through the element deletion algorithm a limit value for the maximum tensile principal stress of ólim=36.5 MPa has been set. All the elements with damage value higher than the critical value will be deleted.

The effects of the die inclination angle on the Maximum Principal stress and on the hole formation mechanism have been analysed.

7. RESULTS

As a first step, the simulation results have been compared with literature and experiments in order to validate the simulation model.

In particular, by analysing the predicted stress distributions a good match with literature has been found. As reported in Figure 3 an “X” shape in the stress distributions can be noticed [2, 3].

A more interesting comparison can be done analysing Figure 5. In fact, it shows a macrography of a transversal section of a bar which was cut from a round bar after a stop of the production. The areas of compression and tension are quite evident and a very good comparison can be drawn with the simulation results reported in the right side of Figure 5. In particular, in the simulation results only the line corresponding to the experiments (left side of Figure 5) are drown so facilitating the comparison.

Figure 5. Macrography of a transversal section of the round bar, comparison with simulation results.

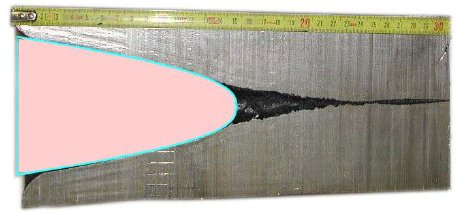
Figure 6. Longitudinal section of the rod, start and development of the hole.

Once the effectiveness of the simulation model has been proved, the attention has been focused on the study of the mechanism of fracture formation and propagation. As first, the effects of the section position in the rolling direction have been analysed while the friction factor was kept constant m=0.8. Referring to Figure 6, which shows the longitudinal section of an experimental round bar it is possible to identify the starting and propagation of the central hole in the rolling direction. The bar comes from the Mannesman process of PIETRA SpA. It is important to point out that the position of the head of the mandrel was, on purpose, kept before the smallest section of the rolls (as shown in Figure 1) in order to have a fracture initiation and propagation not influenced by the mandrel but only due to the stress state exerted by the rolls. The central hole starts to form, as predicted by simulations, far from the mandrel, whose function is only to calibrate the hole once it is developed (see Figure 6). As a consequence, the area under study is the one ahead the mandrel where the hole dimension is determined by the stress state exerted by the rolls. The three die inclination angles, used in the simulation model, have been defined in this area where it is exerted the higher compression of the rod. 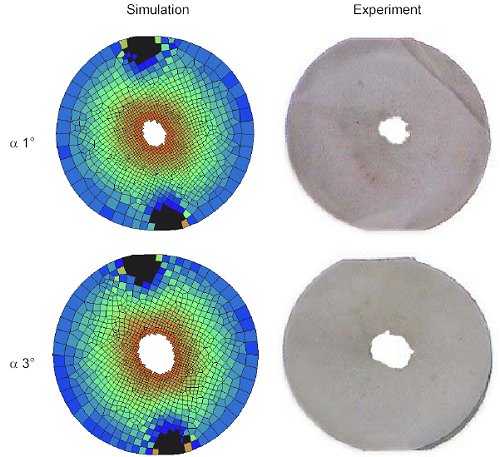The simulation results are presented in Figure 7 for the die inclination angle of 1° and 3°, together with the experimental results. It can be noticed that increasing the tube position in the rolling direction the dimension of the internal hole increases, so representing the starting and the development of the internal hole.

Figure 7. Formation of the internal holein the rolling direction: Comparison between simulation and experiment

The simulation results, in terms of hole dimension and propagation, have been compared with the experimental evidence. In particular, by comparing the plots of the two rows of Figure 7, which refer at the same tube path in the rolling direction, quite a good agreement can be found. It is important to point out again that all the simulations are performed keeping constant the value of the critical stress (ólim=36.5MPa).

8. CONCLUSIONS AND FUTURE WORKS

On the basis of the promising results obtained, the Authors decided to continue to investigate the rotary piercing process. In particular, the attention, for the future, will be focused on the process parameters affecting the central hole formation such as:

• working temperature,
• friction,
• roll geometries,
• mandrel position,
• bar material,..

In this way, a good step in the direction of helping the tube production companies in the understanding of the fundamentals of hole formation and propagation process will be done. Moreover, the cooperation between research and production will give the opportunity to bring the research at a more realistic stage so furnishing the concrete possibility to improve and optimise the production of seamless tubes by using a rotary piercing process.

7. ACKNOWLEDGEMENTS

This work has been made possible thanks to MURST COFIN 2000 funds and to the cooperation of Pietra S.p.A. Company (Brescia - Italy).

8. REFERENCES

1. G. Voswinckel, «Developments in the Field of Piercing Billets for Seamless Tubemaking», internal report of Mannesman.
2. Mori, K. and Osakada, K., 1990, «Finite Element Simulation of Three-Dimensional Deformation in Shape Rolling», Int.J.Numer.Mech.Eng., 30-8 : 1431-1440.
3. Osakada, K., Nakano, J. and Mori, K., 1982, «Finite Element Method for Rigid-Plastic Analysis of Metal Forming - Formulation of Finite Deformation», Int. J. Mech. Sci., 24- 8 :459-468.
4. Oyane, M., Sato, T., Okimoto K. and Shima, S., 1980, «Criteria for Ductile Fracture and Their Applications», J. Mech. Working Technol., 4 : 65-81.
   

   For further information please contact:

   Dipartimento di Ingegneria Meccanica, Università degli Studi di Brescia
   Attn: Elisabetta Ceretti
   - WWW: http://www.unibs.it
   - Email.